MUL 09

[Note: GERMAN versions of the Week 9 tasks are available HERE. I welcome suggestions on how to improve the translations!]

 In this week’s tasks, we look at the multiplicative relation between two numbers (or two quantities) and examine whether or how the relation changes when we add the same amount to both numbers. The underlying idea is similar to that of Week 3, where we investigated the addition strategy, though there the multiplicative relations were not made explicit - students would have had to see the need for such relations themselves.

Monday: Here we consider two ages (expressed in years), where one is 3 times the other. What happens in a year’s time, when both ages will have increased by 1? Clearly, the difference in age stays the same, but viewed multiplicatively, the ages narrow! Burt’s mother is now (a bit) less that 3 times as old as Burt.

Students might use specific values to solve the task, along these lines:
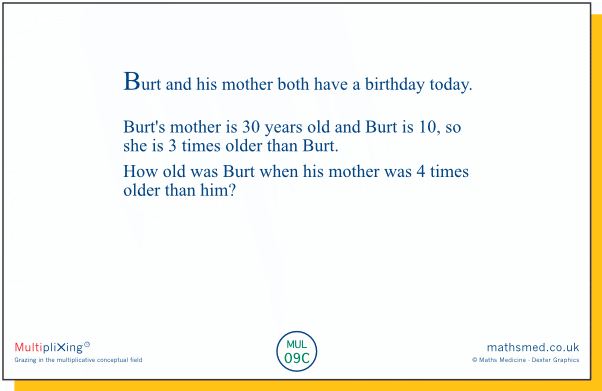
Burt could be 10 years old and his mother 30.
In a year’s time, they would be 11 and 31,
but 31 is less than 3 times 11.
Other students might adopt a general, more insightful approach such as this:
For Burt’s mother to remain 3 times as old as Burt,
her age would have to increase 3 times as much as Burt’s age,
but when Burt is 1 year older, so is she.

Tuesday: Here we reinforce the notion that the multiplicative relation between Burt and his mother's age changes as they get older (by, of course, the same amount).

It turns out that Burt’s mother is 30 and Burt is 10; in 10 years time they will be 40 and 20. Students can approach this in various ways - for example, by trial and improvement, by imagining or drawing a bar diagram, or by expressing the ages algebraically, as here: 3B + 10 = 2(B + 10).

Observation: A teacher might decide to give this task to students before Monday’s task, as it is more straightforward (even if it might take several steps to find the solution). This is perfectly legitimate, but it has its drawbacks: it would deprive students of the opportunity to think more deeply about multiplication that Monday’s task offers, just as it would deprive the teacher of this knowledge about their students’ thinking.
Task-order is an important and interesting issue. In this blog, we sometimes start a weekly set of tasks with a task that is relatively simple, perhaps to introduce students to a new scenario. Sometimes we plunge students in nearer the deep end, to get them to think more independently and deeply, and so reveal more of their ideas including, perhaps, their conceptual difficulties. The benefits of jumping in at the deep end are worth bearing in mind when decisions are made about which tasks to use and in what order.

 

Wednesday: Here we reinforce the notion again that the multiplicative relation between Burt and his mother's age changes as their ages change (by, of course, the same amount). However, the task is more demanding as the solution is not a whole number.

We are told Burt's mother is 3 times as old as Burt now. Do students realise that being 4 times as old must have happened in the past? As the years go by, the multiplicative relation gets closer and closer to ×1. 

The key to the task is that Burt's mother is always 20 years older than Burt. Students using trial and improvement may notice that Burt's mother is 6 times older than Burt when they are 24 and 4, and 5 times older when they are 25 and 5. But for none of the pairs 26 and 6, 27 and 7, 28 and 8, and 29 and 9 is she 4 times as old. (As we know, when we get to 30 and 3 she is only 3 times as old.) So trial and improvement is difficult here. However, students might well be able to find the solution (20÷3 = 6⅔) using a bar diagram or from an equation such as this: B + 20 = 4B. 

Thursday: Here we change to a context of two people sharing a café bill for tea and cake. The payment of £5 and £15 would be ‘fair’ (other things being equal....) if the tea cost nothing. But if the tea is not free, the costs would become more even, so Kim would pay a bit more than £5 and Ken would pay that bit less than £15, and so less than 3 times Kim’s share.
In general terms, when we increase two quantities by the same amount, their relative values get closer, even though the difference between them stays the same. That is essentially what is happening here, as we add the price of the tea to the bill, except that we complicate matters by keeping the bill at £20, which means scaling everything down again. However, this scaling only affects the difference between the shares, not their ratio!

The task is quite challenging, especially if analysed in fairly general terms, as above. One way to make the task more accessible would be to assume that a cup of tea costs the same as a cream cake, in which case Kim and Ken's costs would be in the ratio 2:4 rather than 1:3, resulting in shares of about £6.67 and £13.33 rather than £5 and £15.

Another approach would be to give an 'extreme' value to the price of a cup of tea - £9, say. The cakes would then cost very little (as the bill is still £20), so Kim would pay just under £10 and Ken would pay just over £10 (£9.50 and £10.50).

Or we could assign a more 'normal' value to a cup of tea, say £2, and find the resulting price of a cake [(£20 – £4)÷4], and then calculate the shares accordingly, in this case £6 and £14.

Algebraically, we can express the ratio of Kim's share to Ken's share in the form (t+c):(t+3c) = (a+1):(a+3), where a is the positive number t/c. This ratio will be greater than 1:3 and approaches 1:1 as a get larger.

Friday: Another new context, this time about a wage increase for 10 people in a workshop. In this task, a person whose wage is below the average would gain more from an increase of £10 than an increase of 2%. So if the wage structure is such that most people earn about the same, with one or two earning a lot more (as is often the case), then a £10 increase would probably be more popular. On the other hand, if most earned about the same except for a couple of apprentices, say, then a 2% increase would probably be more popular (unless people had a strong concern for the apprentices’ welfare).

More generally, adding the same amount to everyone's wages will keep the difference between wages the same but will make the ratio between any two wages more equal, ie closer to 1:1. So, to some extent, this levels up. Increasing everyone's wage by the same percentage will increase the difference between wages and keep the ratio the same.

Some students might find it helpful to find the average (mean) weekly wage (£500) and to see what would happen to someone on less than that and to someone on more. For example, for the £10 or 2% increase, someone earning £400 would get £10 or £8, while someone on £600 would get £10 or £12.