In this week's tasks we express amounts of money as fractions of £1, set in the context of the coinage that was in use in the UK before decimalisation in 1971. Decimalisation simplified the currency but we lost some of the nice numerical relations that stemmed, primarily, from the fact that there were 12 (old) pence in one shilling. There are, of course, more factors in 12 than in 10! And a lot more factors in 240 than in 100....
Monday: Here we express each of the 8 coins that were in circulation at the time, as a fraction of £1. You might want to make clear to students that 1 old pence (1d.) does not have the same value as 1 new pence (1p.)

Rather than work through the coins in the order given here (from largest denomination to smallest), you might want to encourage students to reflect on the nature of the fractional relationships by suggesting that they choose an order that they think might be easier. Having found a fraction for one coin, how easily can it be used to find a fraction for another coin?
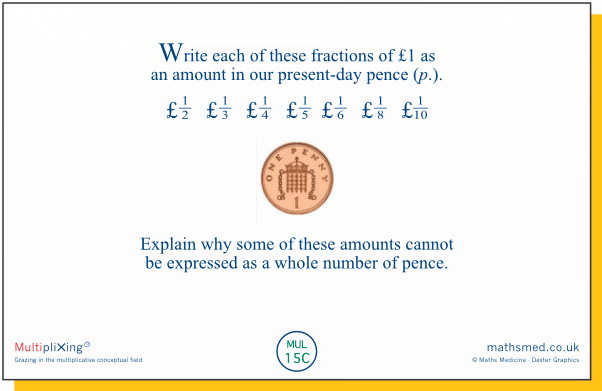
Tuesday: Here we find fractional amounts of £1, using unit fractions from 1/2 to 1/10. It turns out (see Wednesday's task) that more of these amounts can expressed as a whole number of (old) pence (or other coins) than is the case with (new) pence. Why?
Rather than work through the fractional amounts in order, you might want to suggest to students that they choose an order that they think might be easier. Some student might, quite sensibly, express each amount just in pence. If so, ask them to also express the amounts in as few coins as possible. And ask whether they could have found such amounts without expressing them in pence first.
Wednesday: Here is a simpler, neater (?), less interesting (?) version of Tuesday's task. As students will probably have realised by now, 100 has fewer factors than 240!
Thursday: When we work with fractions, rather than thinking of them as pure numbers, we often think of them as parts of a whole (a cake, a bar of chocolate, a rectangle). It can sometimes be helpful to ‘quantify’ this whole (in terms of its price, or weight, or area, etc) and then to work with these quantities rather than with the fractions themselves, before linking the quantities back to fractions again. That is what we do here (and again in Monday’s task of Week 17).

We have given the answers to the three fraction additions shown in this task, to emphasise that our prime aim here is not to perform the computations but to explore (or revisit) a method that may throw light on them. After students have worked through the task, you might want to ask them what other methods could be used to check the answers.
Friday: Here we relate old pence to new pence. The fractions can be derived in a variety of ways. In the case of the first fraction, we could, for example, relate fractions to fractions (⅛ : ⅕); or we could express the numerator and denominator in terms of the same quantity (for example 30d./48d.); or we could transform the fraction into something potentially simpler (for example 5 × 2s.6d. / £1).
Some students might find these fractions quite challenging, in which case ask them to focus on just one - or nominate one for the whole class.